Home » Mathematics » Geometry » Three-Dimensional Figures

Mary Pyo

Mary Pyo

Three-Dimensional Figures

Slide Duration:

Table of Contents

Section 1: Tools of Geometry
Coordinate Plane

16m 41s

Intro
0:00
The Coordinate System
0:12
Coordinate Plane: X-axis and Y-axis
0:15
Quadrants
1:02
Origin
2:00
Ordered Pair
2:17
Coordinate Plane
2:59
Example: Writing Coordinates
3:01
Coordinate Plane, cont.
4:15
Example: Graphing & Coordinate Plane
4:17
Collinear
5:58
Extra Example 1: Writing Coordinates & Quadrants
7:34
Extra Example 2: Quadrants
8:52
Extra Example 3: Graphing & Coordinate Plane
10:58
Extra Example 4: Collinear
12:50
Points, Lines and Planes

17m 17s

Intro
0:00
Points
0:07
Definition and Example of Points
0:09
Lines
0:50
Definition and Example of Lines
0:51
Planes
2:59
Definition and Example of Planes
3:00
Drawing and Labeling
4:40
Example 1: Drawing and Labeling
4:41
Example 2: Drawing and Labeling
5:54
Example 3: Drawing and Labeling
6:41
Example 4: Drawing and Labeling
8:23
Extra Example 1: Points, Lines and Planes
10:19
Extra Example 2: Naming Figures
11:16
Extra Example 3: Points, Lines and Planes
12:35
Extra Example 4: Draw and Label
14:44
Measuring Segments

31m 31s

Intro
0:00
Segments
0:06
Examples of Segments
0:08
Ruler Postulate
1:30
Ruler Postulate
1:31
Segment Addition Postulate
5:02
Example and Definition of Segment Addition Postulate
5:03
Segment Addition Postulate
8:01
Example 1: Segment Addition Postulate
8:04
Example 2: Segment Addition Postulate
11:15
Pythagorean Theorem
12:36
Definition of Pythagorean Theorem
12:37
Pythagorean Theorem, cont.
15:49
Example: Pythagorean Theorem
15:50
Distance Formula
16:48
Example and Definition of Distance Formula
16:49
Extra Example 1: Find Each Measure
20:32
Extra Example 2: Find the Missing Measure
22:11
Extra Example 3: Find the Distance Between the Two Points
25:36
Extra Example 4: Pythagorean Theorem
29:33
Midpoints and Segment Congruence

42m 26s

Intro
0:00
Definition of Midpoint
0:07
Midpoint
0:10
Midpoint Formulas
1:30
Midpoint Formula: On a Number Line
1:45
Midpoint Formula: In a Coordinate Plane
2:50
Midpoint
4:40
Example: Midpoint on a Number Line
4:43
Midpoint
6:05
Example: Midpoint in a Coordinate Plane
6:06
Midpoint
8:28
Example 1
8:30
Example 2
13:01
Segment Bisector
15:14
Definition and Example of Segment Bisector
15:15
Proofs
17:27
Theorem
17:53
Proof
18:21
Midpoint Theorem
19:37
Example: Proof & Midpoint Theorem
19:38
Extra Example 1: Midpoint on a Number Line
23:44
Extra Example 2: Drawing Diagrams
26:25
Extra Example 3: Midpoint
29:14
Extra Example 4: Segment Bisector
33:21
Angles

42m 34s

Intro
0:00
Angles
0:05
Angle
0:07
Ray
0:23
Opposite Rays
2:09
Angles
3:22
Example: Naming Angle
3:23
Angles
6:39
Interior, Exterior, Angle
6:40
Measure and Degrees
7:38
Protractor Postulate
8:37
Example: Protractor Postulate
8:38
Angle Addition Postulate
11:41
Example: Angle addition Postulate
11:42
Classifying Angles
14:10
Acute Angle
14:16
Right Angles
14:30
Obtuse Angle
14:41
Angle Bisector
15:02
Example: Angle Bisector
15:04
Angle Relationships
16:43
Adjacent Angles
16:47
Vertical Angles
17:49
Linear Pair
19:40
Angle Relationships
20:31
Right Angles
20:32
Supplementary Angles
21:15
Complementary Angles
21:33
Extra Example 1: Angles
24:08
Extra Example 2: Angles
29:06
Extra Example 3: Angles
32:05
Extra Example 4 Angles
35:44
Section 2: Reasoning & Proof
Inductive Reasoning

19m

Intro
0:00
Inductive Reasoning
0:05
Conjecture
0:06
Inductive Reasoning
0:15
Examples
0:55
Example: Sequence
0:56
More Example: Sequence
2:00
Using Inductive Reasoning
2:50
Example: Conjecture
2:51
More Example: Conjecture
3:48
Counterexamples
4:56
Counterexample
4:58
Extra Example 1: Conjecture
6:59
Extra Example 2: Sequence and Pattern
10:20
Extra Example 3: Inductive Reasoning
12:46
Extra Example 4: Conjecture and Counterexample
15:17
Conditional Statements

42m 47s

Intro
0:00
If Then Statements
0:05
If Then Statements
0:06
Other Forms
2:29
Example: Without Then
2:40
Example: Using When
3:03
Example: Hypothesis
3:24
Identify the Hypothesis and Conclusion
3:52
Example 1: Hypothesis and Conclusion
3:58
Example 2: Hypothesis and Conclusion
4:31
Example 3: Hypothesis and Conclusion
5:38
Write in If Then Form
6:16
Example 1: Write in If Then Form
6:23
Example 2: Write in If Then Form
6:57
Example 3: Write in If Then Form
7:39
Other Statements
8:40
Other Statements
8:41
Converse Statements
9:18
Converse Statements
9:20
Converses and Counterexamples
11:04
Converses and Counterexamples
11:05
Example 1: Converses and Counterexamples
12:02
Example 2: Converses and Counterexamples
15:10
Example 3: Converses and Counterexamples
17:08
Inverse Statement
19:58
Definition and Example
19:59
Inverse Statement
21:46
Example 1: Inverse and Counterexample
21:47
Example 2: Inverse and Counterexample
23:34
Contrapositive Statement
25:20
Definition and Example
25:21
Contrapositive Statement
26:58
Example: Contrapositive Statement
27:00
Summary
29:03
Summary of Lesson
29:04
Extra Example 1: Hypothesis and Conclusion
32:20
Extra Example 2: If-Then Form
33:23
Extra Example 3: Converse, Inverse, and Contrapositive
34:54
Extra Example 4: Converse, Inverse, and Contrapositive
37:56
Point, Line, and Plane Postulates

17m 24s

Intro
0:00
What are Postulates?
0:09
Definition of Postulates
0:10
Postulates
1:22
Postulate 1: Two Points
1:23
Postulate 2: Three Points
2:02
Postulate 3: Line
2:45
Postulates, cont..
3:08
Postulate 4: Plane
3:09
Postulate 5: Two Points in a Plane
3:53
Postulates, cont..
4:46
Postulate 6: Two Lines Intersect
4:47
Postulate 7: Two Plane Intersect
5:28
Using the Postulates
6:34
Examples: True or False
6:35
Using the Postulates
10:18
Examples: True or False
10:19
Extra Example 1: Always, Sometimes, or Never
12:22
Extra Example 2: Always, Sometimes, or Never
13:15
Extra Example 3: Always, Sometimes, or Never
14:16
Extra Example 4: Always, Sometimes, or Never
15:03
Deductive Reasoning

36m 3s

Intro
0:00
Deductive Reasoning
0:06
Definition of Deductive Reasoning
0:07
Inductive vs. Deductive
2:51
Inductive Reasoning
2:52
Deductive reasoning
3:19
Law of Detachment
3:47
Law of Detachment
3:48
Examples of Law of Detachment
4:31
Law of Syllogism
7:32
Law of Syllogism
7:33
Example 1: Making a Conclusion
9:02
Example 2: Making a Conclusion
12:54
Using Laws of Logic
14:12
Example 1: Determine the Logic
14:42
Example 2: Determine the Logic
17:02
Using Laws of Logic, cont.
18:47
Example 3: Determine the Logic
19:03
Example 4: Determine the Logic
20:56
Extra Example 1: Determine the Conclusion and Law
22:12
Extra Example 2: Determine the Conclusion and Law
25:39
Extra Example 3: Determine the Logic and Law
29:50
Extra Example 4: Determine the Logic and Law
31:27
Proofs in Algebra: Properties of Equality

44m 31s

Intro
0:00
Properties of Equality
0:10
Addition Property of Equality
0:28
Subtraction Property of Equality
1:10
Multiplication Property of Equality
1:41
Division Property of Equality
1:55
Addition Property of Equality Using Angles
2:46
Properties of Equality, cont.
4:10
Reflexive Property of Equality
4:11
Symmetric Property of Equality
5:24
Transitive Property of Equality
6:10
Properties of Equality, cont.
7:04
Substitution Property of Equality
7:05
Distributive Property of Equality
8:34
Two Column Proof
9:40
Example: Two Column Proof
9:46
Proof Example 1
16:13
Proof Example 2
23:49
Proof Example 3
30:33
Extra Example 1: Name the Property of Equality
38:07
Extra Example 2: Name the Property of Equality
40:16
Extra Example 3: Name the Property of Equality
41:35
Extra Example 4: Name the Property of Equality
43:02
Proving Segment Relationship

41m 2s

Intro
0:00
Good Proofs
0:12
Five Essential Parts
0:13
Proof Reasons
1:38
Undefined
1:40
Definitions
2:06
Postulates
2:42
Previously Proven Theorems
3:24
Congruence of Segments
4:10
Theorem: Congruence of Segments
4:12
Proof Example
10:16
Proof: Congruence of Segments
10:17
Setting Up Proofs
19:13
Example: Two Segments with Equal Measures
19:15
Setting Up Proofs
21:48
Example: Vertical Angles are Congruent
21:50
Setting Up Proofs
23:59
Example: Segment of a Triangle
24:00
Extra Example 1: Congruence of Segments
27:03
Extra Example 2: Setting Up Proofs
28:50
Extra Example 3: Setting Up Proofs
30:55
Extra Example 4: Two-Column Proof
33:11
Proving Angle Relationships

33m 37s

Intro
0:00
Supplement Theorem
0:05
Supplementary Angles
0:06
Congruence of Angles
2:37
Proof: Congruence of Angles
2:38
Angle Theorems
6:54
Angle Theorem 1: Supplementary Angles
6:55
Angle Theorem 2: Complementary Angles
10:25
Angle Theorems
11:32
Angle Theorem 3: Right Angles
11:35
Angle Theorem 4: Vertical Angles
12:09
Angle Theorem 5: Perpendicular Lines
12:57
Using Angle Theorems
13:45
Example 1: Always, Sometimes, or Never
13:50
Example 2: Always, Sometimes, or Never
14:28
Example 3: Always, Sometimes, or Never
16:21
Extra Example 1: Always, Sometimes, or Never
16:53
Extra Example 2: Find the Measure of Each Angle
18:55
Extra Example 3: Find the Measure of Each Angle
25:03
Extra Example 4: Two-Column Proof
27:08
Section 3: Perpendicular & Parallel Lines
Parallel Lines and Transversals

37m 35s

Intro
0:00
Lines
0:06
Parallel Lines
0:09
Skew Lines
2:02
Transversal
3:42
Angles Formed by a Transversal
4:28
Interior Angles
5:53
Exterior Angles
6:09
Consecutive Interior Angles
7:04
Alternate Exterior Angles
9:47
Alternate Interior Angles
11:22
Corresponding Angles
12:27
Angles Formed by a Transversal
15:29
Relationship Between Angles
15:30
Extra Example 1: Intersecting, Parallel, or Skew
19:26
Extra Example 2: Draw a Diagram
21:37
Extra Example 3: Name the Figures
24:12
Extra Example 4: Angles Formed by a Transversal
28:38
Angles and Parallel Lines

41m 53s

Intro
0:00
Corresponding Angles Postulate
0:05
Corresponding Angles Postulate
0:06
Alternate Interior Angles Theorem
3:05
Alternate Interior Angles Theorem
3:07
Consecutive Interior Angles Theorem
5:16
Consecutive Interior Angles Theorem
5:17
Alternate Exterior Angles Theorem
6:42
Alternate Exterior Angles Theorem
6:43
Parallel Lines Cut by a Transversal
7:18
Example: Parallel Lines Cut by a Transversal
7:19
Perpendicular Transversal Theorem
14:54
Perpendicular Transversal Theorem
14:55
Extra Example 1: State the Postulate or Theorem
16:37
Extra Example 2: Find the Measure of the Numbered Angle
18:53
Extra Example 3: Find the Measure of Each Angle
25:13
Extra Example 4: Find the Values of x, y, and z
36:26
Slope of Lines

44m 6s

Intro
0:00
Definition of Slope
0:06
Slope Equation
0:13
Slope of a Line
3:45
Example: Find the Slope of a Line
3:47
Slope of a Line
8:38
More Example: Find the Slope of a Line
8:40
Slope Postulates
12:32
Proving Slope Postulates
12:33
Parallel or Perpendicular Lines
17:23
Example: Parallel or Perpendicular Lines
17:24
Using Slope Formula
20:02
Example: Using Slope Formula
20:03
Extra Example 1: Slope of a Line
25:10
Extra Example 2: Slope of a Line
26:31
Extra Example 3: Graph the Line
34:11
Extra Example 4: Using the Slope Formula
38:50
Proving Lines Parallel

25m 55s

Intro
0:00
Postulates
0:06
Postulate 1: Parallel Lines
0:21
Postulate 2: Parallel Lines
2:16
Parallel Postulate
3:28
Definition and Example of Parallel Postulate
3:29
Theorems
4:29
Theorem 1: Parallel Lines
4:40
Theorem 2: Parallel Lines
5:37
Theorems, cont.
6:10
Theorem 3: Parallel Lines
6:11
Extra Example 1: Determine Parallel Lines
6:56
Extra Example 2: Find the Value of x
11:42
Extra Example 3: Opposite Sides are Parallel
14:48
Extra Example 4: Proving Parallel Lines
20:42
Parallels and Distance

19m 48s

Intro
0:00
Distance Between a Points and Line
0:07
Definition and Example
0:08
Distance Between Parallel Lines
1:51
Definition and Example
1:52
Extra Example 1: Drawing a Segment to Represent Distance
3:02
Extra Example 2: Drawing a Segment to Represent Distance
4:27
Extra Example 3: Graph, Plot, and Construct a Perpendicular Segment
5:13
Extra Example 4: Distance Between Two Parallel Lines
15:37
Section 4: Congruent Triangles
Classifying Triangles

28m 43s

Intro
0:00
Triangles
0:09
Triangle: A Three-Sided Polygon
0:10
Sides
1:00
Vertices
1:22
Angles
1:56
Classifying Triangles by Angles
2:59
Acute Triangle
3:19
Obtuse Triangle
4:08
Right Triangle
4:44
Equiangular Triangle
5:38
Definition and Example of an Equiangular Triangle
5:39
Classifying Triangles by Sides
6:57
Scalene Triangle
7:17
Isosceles Triangle
7:57
Equilateral Triangle
8:12
Isosceles Triangle
8:58
Labeling Isosceles Triangle
9:00
Labeling Right Triangle
10:44
Isosceles Triangle
11:10
Example: Find x, AB, BC, and AC
11:11
Extra Example 1: Classify Each Triangle
13:45
Extra Example 2: Always, Sometimes, or Never
16:28
Extra Example 3: Find All the Sides of the Isosceles Triangle
20:29
Extra Example 4: Distance Formula and Triangle
22:29
Measuring Angles in Triangles

44m 43s

Intro
0:00
Angle Sum Theorem
0:09
Angle Sum Theorem for Triangle
0:11
Using Angle Sum Theorem
4:06
Find the Measure of the Missing Angle
4:07
Third Angle Theorem
4:58
Example: Third Angle Theorem
4:59
Exterior Angle Theorem
7:58
Example: Exterior Angle Theorem
8:00
Flow Proof of Exterior Angle Theorem
15:14
Flow Proof of Exterior Angle Theorem
15:17
Triangle Corollaries
27:21
Triangle Corollary 1
27:50
Triangle Corollary 2
30:42
Extra Example 1: Find the Value of x
32:55
Extra Example 2: Find the Value of x
34:20
Extra Example 3: Find the Measure of the Angle
35:38
Extra Example 4: Find the Measure of Each Numbered Angle
39:00
Exploring Congruent Triangles

26m 46s

Intro
0:00
Congruent Triangles
0:15
Example of Congruent Triangles
0:17
Corresponding Parts
3:39
Corresponding Angles and Sides of Triangles
3:40
Definition of Congruent Triangles
11:24
Definition of Congruent Triangles
11:25
Triangle Congruence
16:37
Congruence of Triangles
16:38
Extra Example 1: Congruence Statement
18:24
Extra Example 2: Congruence Statement
21:26
Extra Example 3: Draw and Label the Figure
23:09
Extra Example 4: Drawing Triangles
24:04
Proving Triangles Congruent

47m 51s

Intro
0:00
SSS Postulate
0:18
Side-Side-Side Postulate
0:27
SAS Postulate
2:26
Side-Angle-Side Postulate
2:29
SAS Postulate
3:57
Proof Example
3:58
ASA Postulate
11:47
Angle-Side-Angle Postulate
11:53
AAS Theorem
14:13
Angle-Angle-Side Theorem
14:14
Methods Overview
16:16
Methods Overview
16:17
SSS
16:33
SAS
17:06
ASA
17:50
AAS
18:17
CPCTC
19:14
Extra Example 1:Proving Triangles are Congruent
21:29
Extra Example 2: Proof
25:40
Extra Example 3: Proof
30:41
Extra Example 4: Proof
38:41
Isosceles and Equilateral Triangles

27m 53s

Intro
0:00
Isosceles Triangle Theorem
0:07
Isosceles Triangle Theorem
0:09
Isosceles Triangle Theorem
2:26
Example: Using the Isosceles Triangle Theorem
2:27
Isosceles Triangle Theorem Converse
3:29
Isosceles Triangle Theorem Converse
3:30
Equilateral Triangle Theorem Corollaries
4:30
Equilateral Triangle Theorem Corollary 1
4:59
Equilateral Triangle Theorem Corollary 2
5:55
Extra Example 1: Find the Value of x
7:08
Extra Example 2: Find the Value of x
10:04
Extra Example 3: Proof
14:04
Extra Example 4: Proof
22:41
Section 5: Triangle Inequalities
Special Segments in Triangles

43m 44s

Intro
0:00
Perpendicular Bisector
0:06
Perpendicular Bisector
0:07
Perpendicular Bisector
4:07
Perpendicular Bisector Theorems
4:08
Median
6:30
Definition of Median
6:31
Median
9:41
Example: Median
9:42
Altitude
12:22
Definition of Altitude
12:23
Angle Bisector
14:33
Definition of Angle Bisector
14:34
Angle Bisector
16:41
Angle Bisector Theorems
16:42
Special Segments Overview
18:57
Perpendicular Bisector
19:04
Median
19:32
Altitude
19:49
Angle Bisector
20:02
Examples: Special Segments
20:18
Extra Example 1: Draw and Label
22:36
Extra Example 2: Draw the Altitudes for Each Triangle
24:37
Extra Example 3: Perpendicular Bisector
27:57
Extra Example 4: Draw, Label, and Write Proof
34:33
Right Triangles

26m 34s

Intro
0:00
LL Theorem
0:21
Leg-Leg Theorem
0:25
HA Theorem
2:23
Hypotenuse-Angle Theorem
2:24
LA Theorem
4:49
Leg-Angle Theorem
4:50
LA Theorem
6:18
Example: Find x and y
6:19
HL Postulate
8:22
Hypotenuse-Leg Postulate
8:23
Extra Example 1: LA Theorem & HL Postulate
10:57
Extra Example 2: Find x So That Each Pair of Triangles is Congruent
14:15
Extra Example 3: Two-column Proof
17:02
Extra Example 4: Two-column Proof
21:01
Indirect Proofs and Inequalities

33m 30s

Intro
0:00
Writing an Indirect Proof
0:09
Step 1
0:49
Step 2
2:32
Step 3
3:00
Indirect Proof
4:30
Example: 2 + 6 = 8
5:00
Example: The Suspect is Guilty
5:40
Example: Measure of Angle A < Measure of Angle B
6:06
Definition of Inequality
7:47
Definition of Inequality & Example
7:48
Properties of Inequality
9:55
Comparison Property
9:58
Transitive Property
10:33
Addition and Subtraction Properties
12:01
Multiplication and Division Properties
13:07
Exterior Angle Inequality Theorem
14:12
Example: Exterior Angle Inequality Theorem
14:13
Extra Example 1: Draw a Diagram for the Statement
18:32
Extra Example 2: Name the Property for Each Statement
19:56
Extra Example 3: State the Assumption
21:22
Extra Example 4: Write an Indirect Proof
25:39
Inequalities for Sides and Angles of a Triangle

17m 26s

Intro
0:00
Side to Angles
0:10
If One Side of a Triangle is Longer Than Another Side
0:11
Converse: Angles to Sides
1:57
If One Angle of a Triangle Has a Greater Measure Than Another Angle
1:58
Extra Example 1: Name the Angles in the Triangle From Least to Greatest
2:38
Extra Example 2: Find the Longest and Shortest Segment in the Triangle
3:47
Extra Example 3: Angles and Sides of a Triangle
4:51
Extra Example 4: Two-column Proof
9:08
Triangle Inequality

28m 11s

Intro
0:00
Triangle Inequality Theorem
0:05
Triangle Inequality Theorem
0:06
Triangle Inequality Theorem
4:22
Example 1: Triangle Inequality Theorem
4:23
Example 2: Triangle Inequality Theorem
9:40
Extra Example 1: Determine if the Three Numbers can Represent the Sides of a Triangle
12:00
Extra Example 2: Finding the Third Side of a Triangle
13:34
Extra Example 3: Always True, Sometimes True, or Never True
18:18
Extra Example 4: Triangle and Vertices
22:36
Inequalities Involving Two Triangles

29m 36s

Intro
0:00
SAS Inequality Theorem
0:06
SAS Inequality Theorem & Example
0:25
SSS Inequality Theorem
4:33
SSS Inequality Theorem & Example
4:34
Extra Example 1: Write an Inequality Comparing the Segments
6:08
Extra Example 2: Determine if the Statement is True
9:52
Extra Example 3: Write an Inequality for x
14:20
Extra Example 4: Two-column Proof
17:44
Section 6: Quadrilaterals
Parallelograms

29m 11s

Intro
0:00
Quadrilaterals
0:06
Four-sided Polygons
0:08
Non Examples of Quadrilaterals
0:47
Parallelograms
1:35
Parallelograms
1:36
Properties of Parallelograms
4:28
Opposite Sides of a Parallelogram are Congruent
4:29
Opposite Angles of a Parallelogram are Congruent
5:49
Angles and Diagonals
6:24
Consecutive Angles in a Parallelogram are Supplementary
6:25
The Diagonals of a Parallelogram Bisect Each Other
8:42
Extra Example 1: Complete Each Statement About the Parallelogram
10:26
Extra Example 2: Find the Values of x, y, and z of the Parallelogram
13:21
Extra Example 3: Find the Distance of Each Side to Verify the Parallelogram
16:35
Extra Example 4: Slope of Parallelogram
23:15
Proving Parallelograms

42m 43s

Intro
0:00
Parallelogram Theorems
0:09
Theorem 1
0:20
Theorem 2
1:50
Parallelogram Theorems, Cont.
3:10
Theorem 3
3:11
Theorem 4
4:15
Proving Parallelogram
6:21
Example: Determine if Quadrilateral ABCD is a Parallelogram
6:22
Summary
14:01
Both Pairs of Opposite Sides are Parallel
14:14
Both Pairs of Opposite Sides are Congruent
15:09
Both Pairs of Opposite Angles are Congruent
15:24
Diagonals Bisect Each Other
15:44
A Pair of Opposite Sides is Both Parallel and Congruent
16:13
Extra Example 1: Determine if Each Quadrilateral is a Parallelogram
16:54
Extra Example 2: Find the Value of x and y
20:23
Extra Example 3: Determine if the Quadrilateral ABCD is a Parallelogram
24:05
Extra Example 4: Two-column Proof
30:28
Rectangles

29m 47s

Intro
0:00
Rectangles
0:03
Definition of Rectangles
0:04
Diagonals of Rectangles
2:52
Rectangles: Diagonals Property 1
2:53
Rectangles: Diagonals Property 2
3:30
Proving a Rectangle
4:40
Example: Determine Whether Parallelogram ABCD is a Rectangle
4:41
Rectangles Summary
9:22
Opposite Sides are Congruent and Parallel
9:40
Opposite Angles are Congruent
9:51
Consecutive Angles are Supplementary
9:58
Diagonals are Congruent and Bisect Each Other
10:05
All Four Angles are Right Angles
10:40
Extra Example 1: Find the Value of x
11:03
Extra Example 2: Name All Congruent Sides and Angles
13:52
Extra Example 3: Always, Sometimes, or Never True
19:39
Extra Example 4: Determine if ABCD is a Rectangle
26:45
Squares and Rhombi

39m 14s

Intro
0:00
Rhombus
0:09
Definition of a Rhombus
0:10
Diagonals of a Rhombus
2:03
Rhombus: Diagonals Property 1
2:21
Rhombus: Diagonals Property 2
3:49
Rhombus: Diagonals Property 3
4:36
Rhombus
6:17
Example: Use the Rhombus to Find the Missing Value
6:18
Square
8:17
Definition of a Square
8:20
Summary Chart
11:06
Parallelogram
11:07
Rectangle
12:56
Rhombus
13:54
Square
14:44
Extra Example 1: Diagonal Property
15:44
Extra Example 2: Use Rhombus ABCD to Find the Missing Value
19:39
Extra Example 3: Always, Sometimes, or Never True
23:06
Extra Example 4: Determine the Quadrilateral
28:02
Trapezoids and Kites

30m 48s

Intro
0:00
Trapezoid
0:10
Definition of Trapezoid
0:12
Isosceles Trapezoid
2:57
Base Angles of an Isosceles Trapezoid
2:58
Diagonals of an Isosceles Trapezoid
4:05
Median of a Trapezoid
4:26
Median of a Trapezoid
4:27
Median of a Trapezoid
6:41
Median Formula
7:00
Kite
8:28
Definition of a Kite
8:29
Quadrilaterals Summary
11:19
A Quadrilateral with Two Pairs of Adjacent Congruent Sides
11:20
Extra Example 1: Isosceles Trapezoid
14:50
Extra Example 2: Median of Trapezoid
18:28
Extra Example 3: Always, Sometimes, or Never
24:13
Extra Example 4: Determine if the Figure is a Trapezoid
26:49
Section 7: Proportions and Similarity
Using Proportions and Ratios

20m 10s

Intro
0:00
Ratio
0:05
Definition and Examples of Writing Ratio
0:06
Proportion
2:05
Definition of Proportion
2:06
Examples of Proportion
2:29
Using Ratio
5:53
Example: Ratio
5:54
Extra Example 1: Find Three Ratios Equivalent to 2/5
9:28
Extra Example 2: Proportion and Cross Products
10:32
Extra Example 3: Express Each Ratio as a Fraction
13:18
Extra Example 4: Fin the Measure of a 3:4:5 Triangle
17:26
Similar Polygons

27m 53s

Intro
0:00
Similar Polygons
0:05
Definition of Similar Polygons
0:06
Example of Similar Polygons
2:32
Scale Factor
4:26
Scale Factor: Definition and Example
4:27
Extra Example 1: Determine if Each Pair of Figures is Similar
7:03
Extra Example 2: Find the Values of x and y
11:33
Extra Example 3: Similar Triangles
19:57
Extra Example 4: Draw Two Similar Figures
23:36
Similar Triangles

34m 10s

Intro
0:00
AA Similarity
0:10
Definition of AA Similarity
0:20
Example of AA Similarity
2:32
SSS Similarity
4:46
Definition of SSS Similarity
4:47
Example of SSS Similarity
6:00
SAS Similarity
8:04
Definition of SAS Similarity
8:05
Example of SAS Similarity
9:12
Extra Example 1: Determine Whether Each Pair of Triangles is Similar
10:59
Extra Example 2: Determine Which Triangles are Similar
16:08
Extra Example 3: Determine if the Statement is True or False
23:11
Extra Example 4: Write Two-Column Proof
26:25
Parallel Lines and Proportional Parts

24m 7s

Intro
0:00
Triangle Proportionality
0:07
Definition of Triangle Proportionality
0:08
Example of Triangle Proportionality
0:51
Triangle Proportionality Converse
2:19
Triangle Proportionality Converse
2:20
Triangle Mid-segment
3:42
Triangle Mid-segment: Definition and Example
3:43
Parallel Lines and Transversal
6:51
Parallel Lines and Transversal
6:52
Extra Example 1: Complete Each Statement
8:59
Extra Example 2: Determine if the Statement is True or False
12:28
Extra Example 3: Find the Value of x and y
15:35
Extra Example 4: Find Midpoints of a Triangle
20:43
Parts of Similar Triangles

27m 6s

Intro
0:00
Proportional Perimeters
0:09
Proportional Perimeters: Definition and Example
0:10
Similar Altitudes
2:23
Similar Altitudes: Definition and Example
2:24
Similar Angle Bisectors
4:50
Similar Angle Bisectors: Definition and Example
4:51
Similar Medians
6:05
Similar Medians: Definition and Example
6:06
Angle Bisector Theorem
7:33
Angle Bisector Theorem
7:34
Extra Example 1: Parts of Similar Triangles
10:52
Extra Example 2: Parts of Similar Triangles
14:57
Extra Example 3: Parts of Similar Triangles
19:27
Extra Example 4: Find the Perimeter of Triangle ABC
23:14
Section 8: Applying Right Triangles & Trigonometry
Pythagorean Theorem

21m 14s

Intro
0:00
Pythagorean Theorem
0:05
Pythagorean Theorem & Example
0:06
Pythagorean Converse
1:20
Pythagorean Converse & Example
1:21
Pythagorean Triple
2:42
Pythagorean Triple
2:43
Extra Example 1: Find the Missing Side
4:59
Extra Example 2: Determine Right Triangle
7:40
Extra Example 3: Determine Pythagorean Triple
11:30
Extra Example 4: Vertices and Right Triangle
14:29
Geometric Mean

40m 59s

Intro
0:00
Geometric Mean
0:04
Geometric Mean & Example
0:05
Similar Triangles
4:32
Similar Triangles
4:33
Geometric Mean-Altitude
11:10
Geometric Mean-Altitude & Example
11:11
Geometric Mean-Leg
14:47
Geometric Mean-Leg & Example
14:18
Extra Example 1: Geometric Mean Between Each Pair of Numbers
20:10
Extra Example 2: Similar Triangles
23:46
Extra Example 3: Geometric Mean of Triangles
28:30
Extra Example 4: Geometric Mean of Triangles
36:58
Special Right Triangles

37m 57s

Intro
0:00
45-45-90 Triangles
0:06
Definition of 45-45-90 Triangles
0:25
45-45-90 Triangles
5:51
Example: Find n
5:52
30-60-90 Triangles
8:59
Definition of 30-60-90 Triangles
9:00
30-60-90 Triangles
12:25
Example: Find n
12:26
Extra Example 1: Special Right Triangles
15:08
Extra Example 2: Special Right Triangles
18:22
Extra Example 3: Word Problems & Special Triangles
27:40
Extra Example 4: Hexagon & Special Triangles
33:51
Ratios in Right Triangles

40m 37s

Intro
0:00
Trigonometric Ratios
0:08
Definition of Trigonometry
0:13
Sine (sin), Cosine (cos), & Tangent (tan)
0:50
Trigonometric Ratios
3:04
Trig Functions
3:05
Inverse Trig Functions
5:02
SOHCAHTOA
8:16
sin x
9:07
cos x
10:00
tan x
10:32
Example: SOHCAHTOA & Triangle
12:10
Extra Example 1: Find the Value of Each Ratio or Angle Measure
14:36
Extra Example 2: Find Sin, Cos, and Tan
18:51
Extra Example 3: Find the Value of x Using SOHCAHTOA
22:55
Extra Example 4: Trigonometric Ratios in Right Triangles
32:13
Angles of Elevation and Depression

21m 4s

Intro
0:00
Angle of Elevation
0:10
Definition of Angle of Elevation & Example
0:11
Angle of Depression
1:19
Definition of Angle of Depression & Example
1:20
Extra Example 1: Name the Angle of Elevation and Depression
2:22
Extra Example 2: Word Problem & Angle of Depression
4:41
Extra Example 3: Word Problem & Angle of Elevation
14:02
Extra Example 4: Find the Missing Measure
18:10
Law of Sines

35m 25s

Intro
0:00
Law of Sines
0:20
Law of Sines
0:21
Law of Sines
3:34
Example: Find b
3:35
Solving the Triangle
9:19
Example: Using the Law of Sines to Solve Triangle
9:20
Extra Example 1: Law of Sines and Triangle
17:43
Extra Example 2: Law of Sines and Triangle
20:06
Extra Example 3: Law of Sines and Triangle
23:54
Extra Example 4: Law of Sines and Triangle
28:59
Law of Cosines

52m 43s

Intro
0:00
Law of Cosines
0:35
Law of Cosines
0:36
Law of Cosines
6:22
Use the Law of Cosines When Both are True
6:23
Law of Cosines
8:35
Example: Law of Cosines
8:36
Extra Example 1: Law of Sines or Law of Cosines?
13:35
Extra Example 2: Use the Law of Cosines to Find the Missing Measure
17:02
Extra Example 3: Solve the Triangle
30:49
Extra Example 4: Find the Measure of Each Diagonal of the Parallelogram
41:39
Section 9: Circles
Segments in a Circle

22m 43s

Intro
0:00
Segments in a Circle
0:10
Circle
0:11
Chord
0:59
Diameter
1:32
Radius
2:07
Secant
2:17
Tangent
3:10
Circumference
3:56
Introduction to Circumference
3:57
Example: Find the Circumference of the Circle
5:09
Circumference
6:40
Example: Find the Circumference of the Circle
6:41
Extra Example 1: Use the Circle to Answer the Following
9:10
Extra Example 2: Find the Missing Measure
12:53
Extra Example 3: Given the Circumference, Find the Perimeter of the Triangle
15:51
Extra Example 4: Find the Circumference of Each Circle
19:24
Angles and Arc

35m 24s

Intro
0:00
Central Angle
0:06
Definition of Central Angle
0:07
Sum of Central Angles
1:17
Sum of Central Angles
1:18
Arcs
2:27
Minor Arc
2:30
Major Arc
3:47
Arc Measure
5:24
Measure of Minor Arc
5:24
Measure of Major Arc
6:53
Measure of a Semicircle
7:11
Arc Addition Postulate
8:25
Arc Addition Postulate
8:26
Arc Length
9:43
Arc Length and Example
9:44
Concentric Circles
16:05
Concentric Circles
16:06
Congruent Circles and Arcs
17:50
Congruent Circles
17:51
Congruent Arcs
18:47
Extra Example 1: Minor Arc, Major Arc, and Semicircle
20:14
Extra Example 2: Measure and Length of Arc
22:52
Extra Example 3: Congruent Arcs
25:48
Extra Example 4: Angles and Arcs
30:33
Arcs and Chords

21m 51s

Intro
0:00
Arcs and Chords
0:07
Arc of the Chord
0:08
Theorem 1: Congruent Minor Arcs
1:01
Inscribed Polygon
2:10
Inscribed Polygon
2:11
Arcs and Chords
3:18
Theorem 2: When a Diameter is Perpendicular to a Chord
3:19
Arcs and Chords
5:05
Theorem 3: Congruent Chords
5:06
Extra Example 1: Congruent Arcs
10:35
Extra Example 2: Length of Arc
13:50
Extra Example 3: Arcs and Chords
17:09
Extra Example 4: Arcs and Chords
19:45
Inscribed Angles

27m 53s

Intro
0:00
Inscribed Angles
0:07
Definition of Inscribed Angles
0:08
Inscribed Angles
0:58
Inscribed Angle Theorem 1
0:59
Inscribed Angles
3:29
Inscribed Angle Theorem 2
3:30
Inscribed Angles
4:38
Inscribed Angle Theorem 3
4:39
Inscribed Quadrilateral
5:50
Inscribed Quadrilateral
5:51
Extra Example 1: Central Angle, Inscribed Angle, and Intercepted Arc
7:02
Extra Example 2: Inscribed Angles
9:24
Extra Example 3: Inscribed Angles
14:00
Extra Example 4: Complete the Proof
17:58
Tangents

26m 16s

Intro
0:00
Tangent Theorems
0:04
Tangent Theorem 1
0:05
Tangent Theorem 1 Converse
0:55
Common Tangents
1:34
Common External Tangent
2:12
Common Internal Tangent
2:30
Tangent Segments
3:08
Tangent Segments
3:09
Circumscribed Polygons
4:11
Circumscribed Polygons
4:12
Extra Example 1: Tangents & Circumscribed Polygons
5:50
Extra Example 2: Tangents & Circumscribed Polygons
8:35
Extra Example 3: Tangents & Circumscribed Polygons
11:50
Extra Example 4: Tangents & Circumscribed Polygons
15:43
Secants, Tangents, & Angle Measures

27m 50s

Intro
0:00
Secant
0:08
Secant
0:09
Secant and Tangent
0:49
Secant and Tangent
0:50
Interior Angles
2:56
Secants & Interior Angles
2:57
Exterior Angles
7:21
Secants & Exterior Angles
7:22
Extra Example 1: Secants, Tangents, & Angle Measures
10:53
Extra Example 2: Secants, Tangents, & Angle Measures
13:31
Extra Example 3: Secants, Tangents, & Angle Measures
19:54
Extra Example 4: Secants, Tangents, & Angle Measures
22:29
Special Segments in a Circle

23m 8s

Intro
0:00
Chord Segments
0:05
Chord Segments
0:06
Secant Segments
1:36
Secant Segments
1:37
Tangent and Secant Segments
4:10
Tangent and Secant Segments
4:11
Extra Example 1: Special Segments in a Circle
5:53
Extra Example 2: Special Segments in a Circle
7:58
Extra Example 3: Special Segments in a Circle
11:24
Extra Example 4: Special Segments in a Circle
18:09
Equations of Circles

27m 1s

Intro
0:00
Equation of a Circle
0:06
Standard Equation of a Circle
0:07
Example 1: Equation of a Circle
0:57
Example 2: Equation of a Circle
1:36
Extra Example 1: Determine the Coordinates of the Center and the Radius
4:56
Extra Example 2: Write an Equation Based on the Given Information
7:53
Extra Example 3: Graph Each Circle
16:48
Extra Example 4: Write the Equation of Each Circle
19:17
Section 10: Polygons & Area
Polygons

27m 24s

Intro
0:00
Polygons
0:10
Polygon vs. Not Polygon
0:18
Convex and Concave
1:46
Convex vs. Concave Polygon
1:52
Regular Polygon
4:04
Regular Polygon
4:05
Interior Angle Sum Theorem
4:53
Triangle
5:03
Quadrilateral
6:05
Pentagon
6:38
Hexagon
7:59
20-Gon
9:36
Exterior Angle Sum Theorem
12:04
Exterior Angle Sum Theorem
12:05
Extra Example 1: Drawing Polygons
13:51
Extra Example 2: Convex Polygon
15:16
Extra Example 3: Exterior Angle Sum Theorem
18:21
Extra Example 4: Interior Angle Sum Theorem
22:20
Area of Parallelograms

17m 46s

Intro
0:00
Parallelograms
0:06
Definition and Area Formula
0:07
Area of Figure
2:00
Area of Figure
2:01
Extra Example 1:Find the Area of the Shaded Area
3:14
Extra Example 2: Find the Height and Area of the Parallelogram
6:00
Extra Example 3: Find the Area of the Parallelogram Given Coordinates and Vertices
10:11
Extra Example 4: Find the Area of the Figure
14:31
Area of Triangles Rhombi, & Trapezoids

20m 31s

Intro
0:00
Area of a Triangle
0:06
Area of a Triangle: Formula and Example
0:07
Area of a Trapezoid
2:31
Area of a Trapezoid: Formula
2:32
Area of a Trapezoid: Example
6:55
Area of a Rhombus
8:05
Area of a Rhombus: Formula and Example
8:06
Extra Example 1: Find the Area of the Polygon
9:51
Extra Example 2: Find the Area of the Figure
11:19
Extra Example 3: Find the Area of the Figure
14:16
Extra Example 4: Find the Height of the Trapezoid
18:10
Area of Regular Polygons & Circles

36m 43s

Intro
0:00
Regular Polygon
0:08
SOHCAHTOA
0:54
30-60-90 Triangle
1:52
45-45-90 Triangle
2:40
Area of a Regular Polygon
3:39
Area of a Regular Polygon
3:40
Are of a Circle
7:55
Are of a Circle
7:56
Extra Example 1: Find the Area of the Regular Polygon
8:22
Extra Example 2: Find the Area of the Regular Polygon
16:48
Extra Example 3: Find the Area of the Shaded Region
24:11
Extra Example 4: Find the Area of the Shaded Region
32:24
Perimeter & Area of Similar Figures

18m 17s

Intro
0:00
Perimeter of Similar Figures
0:08
Example: Scale Factor & Perimeter of Similar Figures
0:09
Area of Similar Figures
2:44
Example:Scale Factor & Area of Similar Figures
2:55
Extra Example 1: Complete the Table
6:09
Extra Example 2: Find the Ratios of the Perimeter and Area of the Similar Figures
8:56
Extra Example 3: Find the Unknown Area
12:04
Extra Example 4: Use the Given Area to Find AB
14:26
Geometric Probability

38m 40s

Intro
0:00
Length Probability Postulate
0:05
Length Probability Postulate
0:06
Are Probability Postulate
2:34
Are Probability Postulate
2:35
Are of a Sector of a Circle
4:11
Are of a Sector of a Circle Formula
4:12
Are of a Sector of a Circle Example
7:51
Extra Example 1: Length Probability
11:07
Extra Example 2: Area Probability
12:14
Extra Example 3: Area Probability
17:17
Extra Example 4: Area of a Sector of a Circle
26:23
Section 11: Solids
Three-Dimensional Figures

23m 39s

Intro
0:00
Polyhedrons
0:05
Polyhedrons: Definition and Examples
0:06
Faces
1:08
Edges
1:55
Vertices
2:23
Solids
2:51
Pyramid
2:54
Cylinder
3:45
Cone
4:09
Sphere
4:23
Prisms
5:00
Rectangular, Regular, and Cube Prisms
5:02
Platonic Solids
9:48
Five Types of Regular Polyhedra
9:49
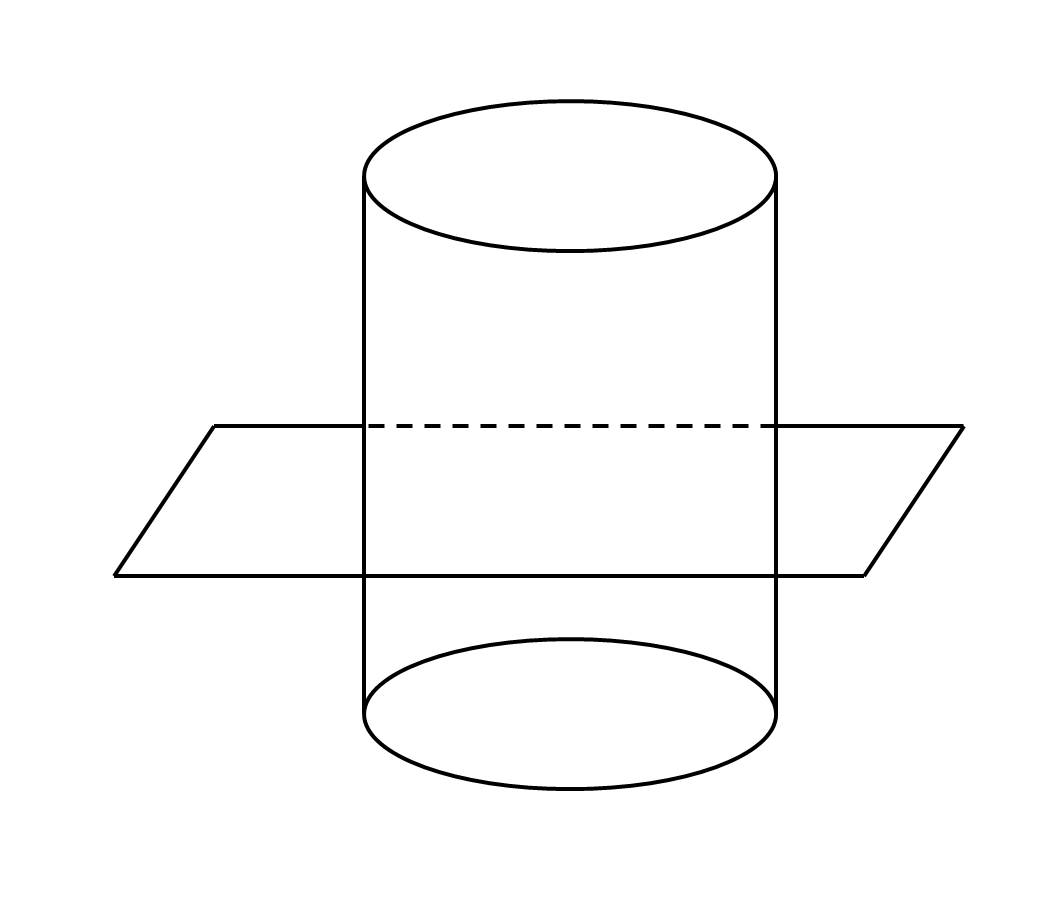
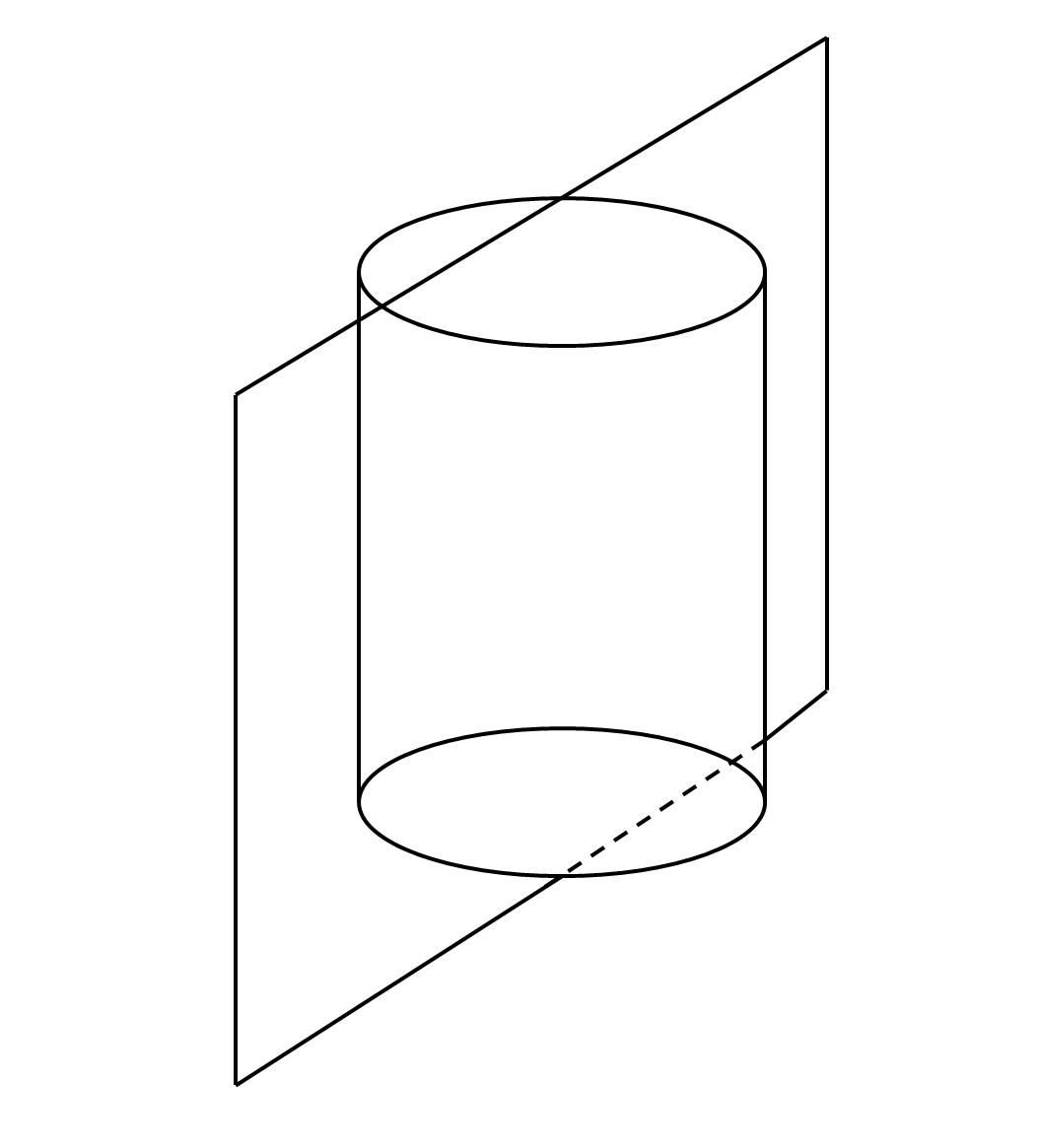
Slices and Cross Sections
12:07
Slices
12:08
Cross Sections
12:47
Extra Example 1: Name the Edges, Faces, and Vertices of the Polyhedron
14:23
Extra Example 2: Determine if the Figure is a Polyhedron and Explain Why
17:37
Extra Example 3: Describe the Slice Resulting from the Cut
19:12
Extra Example 4: Describe the Shape of the Intersection
21:25
Surface Area of Prisms and Cylinders

38m 50s

Intro
0:00
Prisms
0:06
Bases
0:07
Lateral Faces
0:52
Lateral Edges
1:19
Altitude
1:58
Prisms
2:24
Right Prism
2:25
Oblique Prism
2:56
Classifying Prisms
3:27
Right Rectangular Prism
3:28
4:55
Oblique Pentagonal Prism
6:26
Right Hexagonal Prism
7:14
Lateral Area of a Prism
7:42
Lateral Area of a Prism
7:43
Surface Area of a Prism
13:44
Surface Area of a Prism
13:45
Cylinder
16:18
Cylinder: Right and Oblique
16:19
Lateral Area of a Cylinder
18:02
Lateral Area of a Cylinder
18:03
Surface Area of a Cylinder
20:54
Surface Area of a Cylinder
20:55
Extra Example 1: Find the Lateral Area and Surface Are of the Prism
21:51
Extra Example 2: Find the Lateral Area of the Prism
28:15
Extra Example 3: Find the Surface Area of the Prism
31:57
Extra Example 4: Find the Lateral Area and Surface Area of the Cylinder
34:17
Surface Area of Pyramids and Cones

26m 10s

Intro
0:00
Pyramids
0:07
Pyramids
0:08
Regular Pyramids
1:52
Regular Pyramids
1:53
Lateral Area of a Pyramid
4:33
Lateral Area of a Pyramid
4:34
Surface Area of a Pyramid
9:19
Surface Area of a Pyramid
9:20
Cone
10:09
Right and Oblique Cone
10:10
Lateral Area and Surface Area of a Right Cone
11:20
Lateral Area and Surface Are of a Right Cone
11:21
Extra Example 1: Pyramid and Prism
13:11
Extra Example 2: Find the Lateral Area of the Regular Pyramid
15:00
Extra Example 3: Find the Surface Area of the Pyramid
18:29
Extra Example 4: Find the Lateral Area and Surface Area of the Cone
22:08
Volume of Prisms and Cylinders

21m 59s

Intro
0:00
Volume of Prism
0:08
Volume of Prism
0:10
Volume of Cylinder
3:38
Volume of Cylinder
3:39
Extra Example 1: Find the Volume of the Prism
5:10
Extra Example 2: Find the Volume of the Cylinder
8:03
Extra Example 3: Find the Volume of the Prism
9:35
Extra Example 4: Find the Volume of the Solid
19:06
Volume of Pyramids and Cones

22m 2s

Intro
0:00
Volume of a Cone
0:08
Volume of a Cone: Example
0:10
Volume of a Pyramid
3:02
Volume of a Pyramid: Example
3:03
Extra Example 1: Find the Volume of the Pyramid
4:56
Extra Example 2: Find the Volume of the Solid
6:01
Extra Example 3: Find the Volume of the Pyramid
10:28
Extra Example 4: Find the Volume of the Octahedron
16:23
Surface Area and Volume of Spheres

14m 46s

Intro
0:00
Special Segments
0:06
Radius
0:07
Chord
0:31
Diameter
0:55
Tangent
1:20
Sphere
1:43
Plane & Sphere
1:44
Hemisphere
2:56
Surface Area of a Sphere
3:25
Surface Area of a Sphere
3:26
Volume of a Sphere
4:08
Volume of a Sphere
4:09
Extra Example 1: Determine Whether Each Statement is True or False
4:24
Extra Example 2: Find the Surface Area of the Sphere
6:17
Extra Example 3: Find the Volume of the Sphere with a Diameter of 20 Meters
7:25
Extra Example 4: Find the Surface Area and Volume of the Solid
9:17
Congruent and Similar Solids

16m 6s

Intro
0:00
Scale Factor
0:06
Scale Factor: Definition and Example
0:08
Congruent Solids
1:09
Congruent Solids
1:10
Similar Solids
2:17
Similar Solids
2:18
Extra Example 1: Determine if Each Pair of Solids is Similar, Congruent, or Neither
3:35
Extra Example 2: Determine if Each Statement is True or False
7:47
Extra Example 3: Find the Scale Factor and the Ratio of the Surface Areas and Volume
10:14
Extra Example 4: Find the Volume of the Larger Prism
12:14
Section 12: Transformational Geometry
Mapping

14m 12s

Intro
0:00
Transformation
0:04
Rotation
0:32
Translation
1:03
Reflection
1:17
Dilation
1:24
Transformations
1:45
Examples
1:46
Congruence Transformation
2:51
Congruence Transformation
2:52
Extra Example 1: Describe the Transformation that Occurred in the Mappings
3:37
Extra Example 2: Determine if the Transformation is an Isometry
5:16
Extra Example 3: Isometry
8:16
Reflections

23m 17s

Intro
0:00
Reflection
0:05
Definition of Reflection
0:06
Line of Reflection
0:35
Point of Reflection
1:22
Symmetry
1:59
Line of Symmetry
2:00
Point of Symmetry
2:48
Extra Example 1: Draw the Image over the Line of Reflection and the Point of Reflection
3:45
Extra Example 2: Determine Lines and Point of Symmetry
6:59
Extra Example 3: Graph the Reflection of the Polygon
11:15
Extra Example 4: Graph the Coordinates
16:07
Translations

18m 43s

Intro
0:00
Translation
0:05
Translation: Preimage & Image
0:06
Example
0:56
Composite of Reflections
6:28
Composite of Reflections
6:29
Extra Example 1: Translation
7:48
Extra Example 2: Image, Preimage, and Translation
12:38
Extra Example 3: Find the Translation Image Using a Composite of Reflections
15:08
Extra Example 4: Find the Value of Each Variable in the Translation
17:18
Rotations

21m 26s

Intro
0:00
Rotations
0:04
Rotations
0:05
Performing Rotations
2:13
Composite of Two Successive Reflections over Two Intersecting Lines
2:14
Angle of Rotation: Angle Formed by Intersecting Lines
4:29
Angle of Rotation
5:30
Rotation Postulate
5:31
Extra Example 1: Find the Rotated Image
7:32
Extra Example 2: Rotations and Coordinate Plane
10:33
Extra Example 3: Find the Value of Each Variable in the Rotation
14:29
Extra Example 4: Draw the Polygon Rotated 90 Degree Clockwise about P
16:13
Dilation

37m 6s

Intro
0:00
Dilations
0:06
Dilations
0:07
Scale Factor
1:36
Scale Factor
1:37
Example 1
2:06
Example 2
6:22
Scale Factor
8:20
Positive Scale Factor
8:21
Negative Scale Factor
9:25
Enlargement
12:43
Reduction
13:52
Extra Example 1: Find the Scale Factor
16:39
Extra Example 2: Find the Measure of the Dilation Image
19:32
Extra Example 3: Find the Coordinates of the Image with Scale Factor and the Origin as the Center of Dilation
26:18
Extra Example 4: Graphing Polygon, Dilation, and Scale Factor
32:08
Loading...
This is a quick preview of the lesson. For full access, please Log In or Sign up.
For more information, please see full course syllabus of Geometry
Bookmark & Share Embed

Share this knowledge with your friends!

Copy & Paste this embed code into your website’s HTML

Please ensure that your website editor is in text mode when you paste the code.
(In Wordpress, the mode button is on the top right corner.)
  ×
  • - Allow users to view the embedded video in full-size.
Since this lesson is not free, only the preview will appear on your website.

  • Discussion

  • Answer Engine

  • Study Guides

  • Practice Questions

  • Download Lecture Slides

  • Table of Contents

  • Transcription

  • Related Books

Lecture Comments (3)

1 answer

Last reply by: John Stedge
Fri Aug 3, 2018 11:00 AM

Post by Rajendran Rajaram on June 4, 2013

Hey,
I have a question- does a cube and a rectagular prism that has equal surface area have equal volume?

0 answers

Post by Tammy Alvarado on April 30, 2013

im starting to understand solids better thank you mary

Related Articles:

Three-Dimensional Figures

  • Polyhedrons: 3-dimensional solids with all flat surfaces that enclose a single region of space
  • Polyhedrons have faces, edges, and vertices
  • Pyramid: A polyhedron that has all faces expect one intersecting at one point
  • Cylinder: A solid with congruent bases in a pair of parallel planes
  • Cone: A solid with a circular base and a vertex
  • Sphere: A set of points in space that are a given distance from a given point
  • Prism: A polyhedron with two opposite faces parallel and congruent
  • Platonic Solids: The five types of regular polyhedral
  • When a plane intersects, or slice, a solid figure, different shapes are formed
  • If the plane is parallel to the base of the solid, then the intersection of a cross section of the solid

Three-Dimensional Figures

Determine whether the following statement is true or false.
A ball is a polyhedron.
False
Determine whether the following statement is true or false.
All the faces (except the base) of a pyramid expect one intersecting at one point.
True
Fill in the blank in the following statement with sometimes, never or always.
A solid is ________ a polyhedron.
Sometimes
Find what kind of solid is a can of coke look like.
Cylinder
Determine whether the following statement is true or false.
A tetrahedron has four regular faces.
True

Name the edges of the polyhedron.
―AB ,―BC ,―CD ,―AD ,―EF ,―FG ,―GH ,―EH ,―AE ,―BF ,―CG ,―DH

Name the faces of the polyhedron.
ABCD, EFGH, ABFE, BFGC, CGHD, DHEA

Name the vertices of the polyhedron.
A, B, C, D, E, F, G, H

Decribe the cross section of the cylinder.

A circle that is congruent to the base.

Decribe the slice of the cylinder.

A rectangle.

*These practice questions are only helpful when you work on them offline on a piece of paper and then use the solution steps function to check your answer.

Answer

Three-Dimensional Figures

Lecture Slides are screen-captured images of important points in the lecture. Students can download and print out these lecture slide images to do practice problems as well as take notes while watching the lecture.

  • Intro 0:00
  • Polyhedrons 0:05
    • Polyhedrons: Definition and Examples
    • Faces
    • Edges
    • Vertices
  • Solids 2:51
    • Pyramid
    • Cylinder
    • Cone
    • Sphere
  • Prisms 5:00
    • Rectangular, Regular, and Cube Prisms
  • Platonic Solids 9:48
    • Five Types of Regular Polyhedra
  • Slices and Cross Sections 12:07
    • Slices
    • Cross Sections
  • Extra Example 1: Name the Edges, Faces, and Vertices of the Polyhedron 14:23
  • Extra Example 2: Determine if the Figure is a Polyhedron and Explain Why 17:37
  • Extra Example 3: Describe the Slice Resulting from the Cut 19:12
  • Extra Example 4: Describe the Shape of the Intersection 21:25

Transcription: Three-Dimensional Figures

Welcome back to Educator.com.0000

For the next lesson, we are going to go over three-dimensional figures.0002

Another word for three-dimensional figures is solids; now, some specific types of solids are polyhedrons.0007

Whenever you have a three-dimensional solid with all flat surfaces, meaning that all of the sides of that solid are flat, then it is a polyhedron.0018

These are examples of polyhedrons: notice how all of the sides are flat--no round surfaces; no circles,0031

because if you have circles as a side, then the other sides that are intersecting with that are not going to be flat.0042

We are going to go into those types of solids more later.0050

But with these, notice that all of the sides are flat, and it has to enclose a single region of space,0054

meaning that it can't be open; it can't be that one side is missing--it has to be closed.0063

The three parts of polyhedrons: the first one are faces; now, the faces are the sides.0069

All of this right here would be a face, and the same thing with all of this, and this is a face...0080

Each one of those is a face--any time you have a side.0095

This one here has four faces: you have 1, 2, 3, and then the one in the back that you can't see; so each side is a face--these are sides.0099

Edges are the segments that are the intersections of the faces.0116

It is where the faces intersect, so these are all edges here; they are segments.0130

And the vertices are all of these; they are the corners, the endpoints of the edges; so these are like corners, the corners of these polyhedrons.0146

Going over solids: the pyramid is the first one: we know that it is a polyhedron, because all of the sides are flat surfaces.0174

So, all of the faces and one base...the base is the bottom part of this pyramid, so if I draw that part,0186

it is considered the base, the bottom part; and each of these sides here is a lateral face.0205

And all of those faces meet at a point, and that is the vertex.0217

We are going to go over each of these more specifically later on.0222

The next is a cylinder; a cylinder is like a can, like a can of soup; it is a cylinder.0226

The bases would be the circles on top and the bottom, so that would also be the base.0236

A cone is one circular base, and a vertex is right here; think of an ice-cream cone.0252

A sphere is like a ball; and this is not called a circle, because circles are two-dimensional; this is a sphere--it is three-dimensional.0265

A basketball would be a sphere; it is a set of points in space that are a given distance from a given point.0279

This point right here is the center of the sphere; and no matter which way you go, no matter which direction, it is always going to be the same distance.0286

The next type of solids is a prism; a prism is when you have two bases that are opposite and congruent, and they are parallel.0300

This is called a rectangular prism; in this case, I can name any two sides bases,0315

because as long as the two opposite sides are parallel and congruent, they would be the bases of a prism.0325

Now, another example of a prism would be like this; now, if you notice, this is not rectangular.0337

So, this would be the base, and this would be the base opposite; these two bases are parallel and congruent, so this is a prism.0366

Now, the base itself is not a rectangle...this would be called a rectangular prism, because the base is rectangular in shape;0382

and for this one, this is a special type of prism because, if I choose this as the base,0398

the side opposite is also going to be parallel and congruent, so these two can be named the base.0409

Or if I want, I can make the top and the bottom the base, or I can make the front and the back the base,0423

because, as long as, in your prism, you have two opposite faces that are parallel and congruent, they can be the bases of your prism.0435

And that would be the type of prism.0446

Two of the faces are bases; the rest of the faces are lateral faces.0451

So in this one, this top and bottom are your bases; the rest of the sides (that means this one right here,0458

this one right here, the one in the back, and then the three other sides) would be your lateral faces.0466

So, bases and lateral faces make up all of the sides, or faces, of the prism.0474

A regular prism is when the bases of your prism...so then, for this one, if I am going to name0483

the top and the bottom the base, they have to be regular.0493

So, as long as the bases are regular (and regular, again, means equilateral and equiangular), so if I am going to call0497

the top and the bottom the bases, if all of these sides are congruent,0516

and all of the angles are congruent, then this would be considered a regular prism.0521

For this one, if this pentagon (because that is the shape of the base) was regular, then this would be considered a regular prism.0531

And if this is regular, then we know that the bottom base is regular, because they are the same.0545

If the base is a regular polygon, then the whole thing, the prism, is a regular prism.0552

And a cube is when each side of a rectangular prism is a square; so that means the front is a square;0559

the top side is a square; the side is a square...if all of these are squares, then this is a cube.0572

Platonic solids: there are five types of platonic solids, and they are all regular polyhedra.0590

"Polyhedra": if we have one, it is a polyhedron; but when we have more than one, it can be either polyhedrons, with an s to make it plural, or polyhedra.0599

So here are the five types: this one right here has 4 sides, the front, this side...there is a back side, and there is the bottom base.0616

So, it will look like this: 1, 2...and then this is the back side that you can't see...0630

So, the one with four sides (and again, this is all regular, meaning that the sides are all regular) is a tetrahedron.0646

The one with 6 (this is a cube, the one with 6 sides) is a hexahedron.0667

This one has 8 regular sides, so this is an octahedron, just like an octagon.0679

This one has 12 sides, so this is a dodecahedron.0693

This one has 20 sides, and that is an icosahedron; those are the five types of regular polyhedra.0707

OK, slices and cross-sections: whenever you have a solid, and you have a plane that intersects the solid, then you have a slice.0728

"Slice" sounds like you are cutting through, so it is intersecting; so here is a cone, and here is a plane intersecting the cone.0743

See how it is cutting through it...so that is a slice.0752

Then, where they intersect, that is the shape that is formed.0759

Now, if you have the plane intersecting the solid so that the plane is parallel to the base0768

(in this case, the plane is parallel to the base; they are both horizontal, so the base is flat like this,0778

and then the plane is flat or horizontal, cutting through somewhere in the middle), then that would be a cross-section.0786

So, when it is parallel, when the parallelogram is slicing so that it is parallel to the base, then it is a cross-section.0798

So in this case, this would be a cross-section.0808

And the figure, or the shape, that is formed by the intersection would be a circle.0811

Now, you can see here that it is like this; that is where they are intersecting.0817

But that is because it is kind of sideways; if you were to draw it from a top view,0823

meaning if you had a counter right here and you are looking down this way, then you would see a perfect, round circle.0827

That is not perfect, but it is a circle; so that is the shape that resulted from the slice, from the intersection of the plane and the cone.0835

So then, again, when the plane intersects the solid, it is slicing it, and the shape that resulted from that is a circle.0848

Moving on to our examples: name the edges, bases, and vertices of the polyhedron.0865

Remember: edges are all of the sides, all of the segments where all of the faces intersect.0873

So, for the edges, I have to just name all of these segments; so I have AB; I have AE; let me just do that,0884

so that I know that I wrote it down; AE, and then there is that one, BE, this one, AF, BC, ED0905

(it doesn't matter if you do ED or DE), CD, CF, and FD; so then, I have 1, 2, 3, 4, 5, 6, 7, 8, 9--those are all of my edges.0928

And then, bases would be triangle ABE and then the triangle CFD.0959

Then, I am going to name this side here (that is ABCF), then this one here, which is AFDE, and (which one?) this one right here on this side; that is BEDC.0985

Make sure that, when you label it, you label it in order: BEDC--it can't be BDCE--it can't go out of order (but you can do BCDE).1014

And then, we have vertices: vertices would be just these corners, so it would be vertex A, vertex E, vertex B, vertex F, vertex C, and D.1029

Those are the edges, faces, and vertices.1053

The next example: Determine if the figure is a polyhedron, and explain why.1058

This is a cone; it has one base, which is a circle, and we have a vertex.1064

Now, this is a solid, because it is three-dimensional; but it is not a polyhedron, so this would be "no," because polyhedrons have only flat surfaces.1074

Now, this base is flat; it is a flat circle; but how about the cone--isn't it circular? It is not flat.1094

There is no side to this, so it is "no," because it is not a flat surface.1105

So, the solid, because there are no faces, does not have flat surfaces as the sides.1116

Even though, again, the bottom one, the base, is flat, the side here is not flat; it is going circularly.1140

OK, the next example: Describe the slice resulting from the cut.1154

As you can see here, this cone is being cut, or sliced, sideways, like this; so the parallelogram is cutting into the side.1159

Now, what is the shape that has resulted from this cut--what is that cut going to look like, where they intersect?1172

Well, if you have a cone here, it is going to go like this; it is cutting right there--that is a part of it;1184

and then, what about the other side?--it is probably going to go like that, something like this.1197

So, this shape would be a parabola; it is going to be like a U--something like that; that is the shape that is from the slice,1207

from the intersection of the plane and the cone.1224

Just another example: if you have, let's say, a cylinder, and let's say you have a plane that is intersecting,1232

or slicing, that cylinder; well, what is the shape that resulted from the slice?1246

Again, if you look at it from a top view, it is going to be a circle.1261

Think of a can, and you cut in half down the middle; you get a knife, and you just cut it through the middle; aren't you going to get a perfect circle?1272

So, that is another example of a slice.1280

Describe the shape of the intersection: A cube is intersected by a plane that is parallel to the base.1288

A cube is being intersected so that it is parallel to the base; so then, my plane will be something like that.1298

It is being intersected right here; and it is parallel to the base, so that would be a perfect square.1328

A sphere is intersected by a plane at the center of the sphere.1349

I have a sphere; I don't know how you would show a sphere; and it is being intersected right here by a plane.1355

As a result, it is going to look like this; and it has to cut through the center, so what is the result from this slice?1381

It would be a perfect circle, like that.1395

Again, if you look at it from your screen, you are going to see that it is going to look like this; but you have to look at it from a top view.1401

And it is going to be a perfect circle.1412

And that is it; thank you for watching Educator.com.1416

Related Books

Related Books

Educator®

Please sign in to participate in this lecture discussion.

Resetting Your Password?
or
Still having trouble? Contact us
OR

Start Learning Now

Our free lessons will get you started (Adobe Flash® required).
 Get immediate access to our entire library.

Membership Overview

  • Available 24/7. Unlimited Access to Our Entire Library.
  • Search and jump to exactly what you want to learn.
  • *Ask questions and get answers from the community and our teachers!
  • Practice questions with step-by-step solutions.
  • Download lecture slides for taking notes.
  • Track your course viewing progress.
  • Accessible anytime, anywhere with our Android and iOS apps.